The end product of the cotton fiber-to-yarn conversion system is a spun yarn or a staple-fiber yarn, which is suitable for making numerous end products from knit apparels to woven fabrics, from towels to sheets, and from carpets to industrial fabrics. The diversity of yarn-based products results in different views of what constitutes a yarn quality. Indeed, different textile manufacturers often express different views of yarn quality depending on the particular end product produced and the type of downstream processing used.
In general, the spinner may define yarn quality as an index of appearance, strength, uniformity, and level of imperfections. However, the spinner is much more concerned about how the yarn user views yarn quality.
The knitter may have more detailed criteria of yarn quality. These may include:
· A yarn that can unwind smoothly and conform readily to bending and looping while running through the needles and sinkers of the knitting machine. This translates to flexibility and pliability.
· A yarn that sheds low fly in and around the knitting machine. This translates to low hairiness and low fiber fragment content
· A yarn that leads to a fabric of soft hand and comfortable feeling. This translates to low twist, low bending stiffness, and yarn fluffiness or bulkiness.
· A yarn that has better pilling resistance. This translates to good surface integrity.
The weaver may have a different set of yarn quality criteria:
· A yarn that can withstand stresses and potential deformation imposed by the weaving process. This translates to strength, flexibility, and low strength irregularity.
· A yarn that has a good surface integrity. This translates to low hairiness and high abrasion resistance.
· A yarn that can produce defect-free fabric. This translates to high evenness, low imperfection, and minimum contamination.
In light of these different and often conflicting views of yarn quality, the spinner must customize the yarn to meet its intended purpose. This can be achieved through integration of yarn quality into the overall specification of the end product. This requires establishing appropriate values of fiber attributes and optimum machine settings. In an ideal Fiber-to-Yarn Engineering (FYE) program, the translation of yarn quality into an acceptable end product performance is based on a well-defined design approach in which all phases of FYE are integrated to produce a yarn that is consistent and reflective of the end user requirements. This calls for an in-depth knowledge, not only of the general yarn characteristics, but also of the structural features of yarn.
Basic Structural Features of Spun Yarn
Before proceeding with the discussion on yarn characteristics, it will be important to discuss the basic structural features of spun yarn. Understanding these features provides an insight into the interpretation of yarn behavior during processing or in the end product. The basic structural features of spun yarn are: yarn density, bulk integrity, and surface profile. These features are discussed below.
Yarn Density
In a yarn structure, fibers represent the main component. The other component is air pockets created by the technology forming the structure. Accordingly, the yarn bulk density should be determined by the packing fraction, 0, as defined by the following equation:

The packing fraction is an indication of the air spaces enclosed by the fibers. For example, a packing fraction of 0.5 indicates that there is as much space taken by air as by fiber. Most spun yarns have packing fraction well above 0.5.
The importance of packing fraction lies in its powerful effects on many yarn and fabric properties. It is indeed one of the major design parameters of textile fabrics. For a given fiber material, a yarn of very high packing fraction is likely to be stiff and probably weak. On the other hand, a yarn of very low packing fraction is likely to lack the bulk and surface integrity required to hold the yarn structure together during processing.
In relation to fabric performance, yarn density plays a major role in determining many of the performance characteristics of fabric. One of the major fabric characteristics influenced by yarn density is fabric comfort. In general, fabric comfort is viewed in terms of two main aspects [El Mogahzy, 1998]: neurophysiological and thermo-physiological comfort. The neuro-physiological aspect deals with the fabric/skin physical interaction, and the thermo-physiological aspect deals with moisture and heat transfer through fabric. A high packing fraction will likely produce a highly compacted yarn that is likely to produce a stiff fabric and result in a greater true contact between the fabric and the human skin. These two features typically result in neuro-physiological discomfort. The thermo-physiological effect can be explained on the ground that air is the best heat insulator of all materials. On average, the thermal conductivity of air is more than eight times less than that of fibers (thermal conductivity of air = 6x10-5 cal.sec -1.cm -1. deg -1 C). The air pockets in the yarn assists in creating an entrapped or still air in the fabric and this can greatly enhance the thermal insulation of the human body against changing environmental conditions. Yarn density also influences other characteristics such as dimensional stability, strength, extensibility, flexibility, fabric cover, air permeability, and absorption characteristics.
Staple fiber yarns and textured yarns normally have lower density than continuous filament yarns made from the same fiber material. As will be discussed in chapter 9, different spinning techniques produce different degrees of yarn density as a result of the different patterns of fiber compactness imposed by yarn twisting and spinning tension. For instance, a ring-spun yarn will typically exhibit higher degree of compactness than a comparable rotor-spun yarn due to the true twist the high tension used in ring spinning. The extent of fiber compactness can also be altered within the same spinning system. For instance, a higher rotor speed in open-end spinning is likely to produce higher fiber compactness in the yarn due to the higher centrifugal force applied on the fibers inside the rotor. The introduction of compact ring spinning has resulted in better compactness of fibers in the yarn as will be shown in Chapter 9.
In theory, yarn density has approximately linear relationship with the product (twist.tex1/4) of spun yarn (Neckar, 1998). Fiber properties that influence this product will also influence fiber compactness or yarn density. These include: fiber diameter, cross-sectional shape, fiber length, fiber resiliency, and fiber density. For a given yarn count, and a given twist level, fine and long fibers will normally result in higher yarn density than coarse and short fibers.
Yarn Bulk Integrity
Yarn bulk integrity is determined by the fiber arrangement in the yarn structure. Fiber arrangement is expected to have significant effects on many yarn and fabric characteristics including yarn liveliness, fabric dimensional stability, yarn appearance, yarn strength, and fabric cover. The bulk integrity of a spun yarn largely reflects the impact of the spinning process on yarn structure. In general, different spinning techniques provide different forms of bulk integrity through providing different fiber arrangements. Obviously, the simplest fiber arrangement can be found in a continuous filament yarn where fibers (or continuous filaments) are typically arranged in parallel and straight form. As shown in Figure 7. 1, a slight deviation from this arrangement can be caused by slightly twisting the filaments or through deliberate distortion in the filament orientation as in the texturizing process.
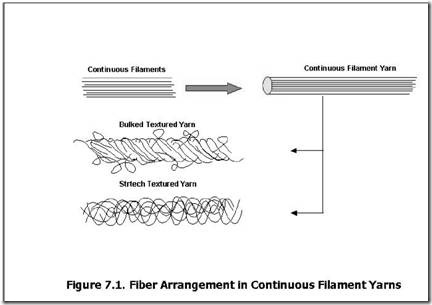
In staple fiber yarns, fiber arrangement is quite different from the simple arrangement discussed above. The discrete nature of staple fibers makes it impossible to fully control the fiber flow in such a way that can produce a well-defined fiber arrangement. For this reason, a spun yarn typically exhibits some irregularities along the yarn axis. In addition, no spun yarn can be free of fiber ends protruding from its surface as shown in Figure 7.2. Different spinning systems produce different forms of bulk integrity or fiber arrangements. The general features of fiber arrangement produced by four different spinning systems are shown in Figure 7.3 shows. In chapter 9, we will discuss how these spinning techniques produce these structural arrangements.

Yarn Surface Profile
The surface profile of a spun yarn may be described by three basic parameters: the overall surface appearance of yarn, surface integrity, and surface irregularities. The importance of yarn surface profile lies in the fact that a yarn is initially judged by its surface appearance. As the yarn goes through the weaving or the knitting process, surface integrity (abrasion resistance and hairiness) becomes the most critical factor determining yarn performance. As the yarn is finally woven or knitted into a fabric, surface irregularities (thick and thin places, and yarn neps) are typically the most noticeable defects in the fabric.
As expected, yarn density (or fiber compactness), and bulk integrity (or fiber arrangement) will greatly influence yarn surface profile. Accordingly, different spinning techniques will produce yarns of different surface profiles. Within a given spinning system, the main factors influencing yarn surface profile are:
· The drafting mechanism (roller drafting or aerodynamic drafting)
· The consolidation mechanism (twisting or wrapping)
· The surface roughness of the spinning component (e.g. the traveler/ring contact in ring-spinning, the navel surface in rotor-spinning, and the condensation surface in compact spinning)
Material-related factors that can influence yarn surface profile include:
· Short fiber content
· Fiber neps
· Fiber rigidity (flexural and torsion rigidities)
· Fiber contaminants
In general, high levels of short fibers can result in excessive surface hairiness particularly in ring-spun yarns. The fact that short fibers flow under minimum or no control in the textile process can result in many surface disturbances of yarns spun on any spinning system. Many of the random thick and thin places in the yarn can be attributed to short fibers. Fiber neps that are not removed by the textile process will be presented in the yarn either in the bulk or on the surface. In both cases, surface disturbances will appear as short thick places or yarn neps. Fibers of high bending or torsion rigidity will not conform to the manipulation process exerted during textile processing. These fibers are likely to act in an unpredictable fashion leading to many surface disturbances (hairiness or irregular fiber wrappers). Many of the long-fiber hairiness can be attributed to high rigidity. On the other hand, fibers of extremely low rigidity may tend to entangle and form neps. Extraneous materials typically exhibit different shapes, colors, and sizes from fibers. The chance of these contaminants to appear on the yarn surface is much greater than to be incorporated in the yarn bulk. In either case, these contaminants, if not removed in the early stage of processing, can alter the yarn surface profile substantially.
Yarn Characteristics
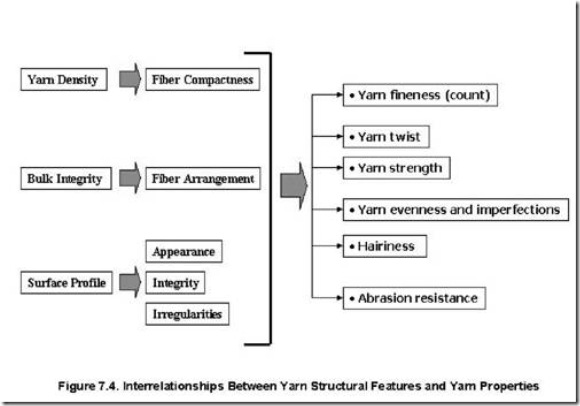
The basic structural features discussed above collectively determine the different yarn characteristics that we routinely measure in practice (see Figure 7.4). These include:
· Yarn fineness (count)
· Yarn twist
· Yarn strength
· Yarn evenness and imperfections
· Surface integrity (hairiness and abrasion resistance)
In the following sections, we will briefly discuss these characteristics.
Yarn Fineness or Count
In practice, yarn fineness is typically described by terms such as yarn count, yarn number, or yarn size. The subject of yarn fineness can be treated in a similar manner to that of fiber fineness in the sense that both the fiber and the yarn may not have perfectly circular cross sections and they both exhibit thickness variability. Therefore, the linear density or mass per unit length is commonly used as an alternative measure of actual fineness or thickness. In general, two yarn count systems are commonly used: (i) the direct system, and (ii) the indirect system.
Direct Count System
In a direct system, yarn count is the mass of a unit length of yarn. One of the universally used direct systems is known as the "tex". This is defined by the mass in grams of 1 km of yarn. Both the millitex and the decitex mentioned earlier for fibers are extensions of the tex system. For intermediate heavy products such as slivers, the "kilotex" is commonly used. This is the mass in kilograms per kilometer (or equivalently, grams per meter). A more common direct system for slivers is the grains/yd, where a grain is 1/7000lb. For continuous filament yarns, the denier system is used; this is the weight in grams of 9,000 meters.
Indirect Count System
In an indirect system, the yarn number or count is expressed in "units of length" per "unit of weight". Several indirect systems are used in practice depending on the type of yarn produced, and the spinning system. For cotton yarns, the "English" or "cotton" count is used to express yarn fineness. The unit of length in an "English" count system is the hank, 840 yd, and the unit of weight is 1 lb. Normally, yarn count is determined by determining the mass of 120 yd of yarn. For example, if the weight of a 120 yd yarn
is 0.004 lb, the English or cotton count will be 120/(840x0.004), or 35.7. In symbols, this is commonly written as Ne = 35.7's. The cotton count may also be used for heavier products such as slivers.
For wool yarns, two indirect systems are commonly used: (i) the Woolen system, and (ii) the Worsted system. Within the Woolen system, several sub-systems are utilized. These include: (a) the Woolen (American cut) with a unit length of 300 yards cut, and the unit of weight is pound, and (b) the Woolen (American run) with a unit length of 100 yards, and the unit of weight is ounce. In the Worsted system, the unit length (or the hank) is 560 yards, and the unit of weight is pound.
Another indirect system is the "metric" system commonly used in Europe. In this system, the unit length is kilometer, and the unit of mass is kg. The conversion from one count system to another is a simple mathematical task. Table 7.1 gives a number of conversion constants, which can be used to convert from one system to another.

Plied Yarns
We can make a plied yarn by twisting two single yarns together (Figure 7.5). The resultant yarn count is calculated as follows:
Using a direct count system:

Normally, when two single yarns are twisted together one should expect some contraction or some increase in length depending on the twisting direction. A contraction will result in a yarn count slightly coarser than the estimated value. In order to correct for this difference, typically 5% to 10% contraction or extension should be accounted for. This point will be explained shortly.

Count Variation: C.Vcount ~
Variation in yarn count is as important as the average value of count. High-count variation can result in many quality problems including: high yarn irregularity, variation in fabric weight, and variation in dye
uptake or barre. Count variation is defined by:


Yarn Twist
Twisting is the primary binding mechanism of spun yarns. In general, twist is defined as a measure of spiral turns given to a yarn in order to hold the constituent fibers together. In practice, yarn twist is described using three main parameters: (a) twist direction, (b) twist level (turns/unit length), and (c) twist factor.
Twist Direction
Twist may be performed in the following two directions (Figure 7.6):
S-Direction: A single yarn has "S" twist if, when it is held in the vertical position, the fibers inclined to the axis of the yarn conform in direction of slope to the central portion of the letter S.
Z-Direction: A single yarn has "Z" twist if, when it is held in the vertical position, the fibers inclined to the axis of the yarn conform in direction of slope to the central portion of the letter Z.

Twist Level
The amount of twist in the yarn is commonly expressed by the number of turns per unit length. In order to understand the meaning of twist and its relation to other yarn parameters, we will use the classical idealized helical geometry of a circular yarn (Hearle et al, 1969) shown in Figure 7.6. In this geometry, the yarn is assumed to be built-up of a series of superimposed concentric layers of different radii in each of which the fibers follow a uniform helical path so that its distance from the center remains constant. Based on this model, the length of one turn of twist, h, is given by:


where TPC is turns per cm, and TPI is turns per inch.
In practice, equation 7.11 is commonly used to determine the twist multiplier of yarn for a given yarn count and a given twist level. It simply indicates that the twist multiplier is an expression of the twist level adjusted for yarn count.
The Importance of Yarn Twist
In practice, the importance of twist direction is realized when two single yarns are twisted to form a ply yarn. Ply twist may be Z on Z, or S on Z depending on appearance and strength requirements of the ply yarn. Recall that in determining the yarn count of a plied yarn, we had to account for the possible contraction or increase in length resulting from twisting. Normally, the Z on Z twist will result in a contraction of the plied yarn, while the S on Z twist will result in an increase in length. This amount of contraction or expansion will depend on the amount of twist inserted.
When the yarn is woven or knitted into a fabric, the direction of twist influences the appearance of fabric. When a cloth is woven with the warp threads in alternate bands of S and Z twist, a subdued stripe effect is observed in the finished cloth due to the difference in the way the incident light is reflected from the two sets of yarns. In twill fabric, the direction of twist in the yarn largely determines the predominance of twill effect. For right-handed twill, the best contrasting effect will be obtained when a yarn with Z twist is used; on the other hand, a left-handed twist will produce a fabric having a flat appearance. In some cases, yarns with opposite twist directions are used to produce special surface texture effects in crepe fabrics.
Twist direction will also have a great influence on fabric stability, which may be described by the amount of skew or "torque" in the fabric. This problem often exists in cotton single jersey knit where knitted wales and courses are angularly displaced from the ideal perpendicular angle. One of the solutions to solve this problem is to coordinate the direction of twist with the direction of machine rotation. With other factors being similar, yarn of Z twist is found to give less skew with machines rotating counterclockwise. Fabrics coming off the needles of a counterclockwise rotating machine have courses with left-hand skew, and yarns with Z twist yield right-hand wale skew. Thus, the two effects offset each other to yield less net skew. Clockwise rotating machines yield less skew with S twist.
The amount of twist inserted in the yarn can influence many yarn characteristics. As will be shown in chapter 9, twisting is the primary mechanism to bind fibers in both ring and open-end spinning. Twisting is a unique binding mechanism that many engineers outside the textile field are not familiar with. It is, perhaps, the only binding mechanism that allows the structure to retain a great deal of its flexibility (as compared to glue or adhesive chemicals which result in more stiff structures).
The relationship between yarn strength and twist level is well recognized among textile technologists and engineers. This relationship is generally illustrated in Figure 7.7. Initially, as the twist level (number of turns per unit length) increases, yarn strength will also increase. This effect holds only up to a certain point beyond which further increase in twist causes the yarn to become weaker. Thus, one should expect a point of twist at which yarn strength is at its maximum value. This point is known as the "optimum twist".

Many investigators made various attempts to explain the strength-twist relationship (e.g. Hearle et al, 1969, and Lord, 1981). In practical terms, the strength-twist relationship may be explained on the ground that at zero twist, fibers are more or less oriented along the yarn axis but without any binding forces (except their interfacial contact). As twist slightly increases, the contact between fibers will increase due to the increase in traverse pressure, and the force required to stretch the yarn must first overcome the inter-fiber friction. Further increase in twist will result in further binding between fibers and an increase in the number of cross-linking points between fibers. This provides an opportunity for many fibers to be held at some points along their axis by other fibers. When this happens, the fiber strength begins to play a role in resisting the force required to stretch or rupture the yarn. Eventually, fiber strength will play a greater role than interfiber friction in tensile resistance. However, the discrete nature of fibers will always necessitate inter-fiber cohesion. The trend of increasing strength with twist will continue until some points where the fibers become so inclined away from the yarn axis that the contribution of fiber strength will decrease. This will result in a reduction of yarn strength with the increase in twist.
In light of the above interpretation, one can see that there are two effects governing the strength-twist relationship. The first effect is an increase in yarn strength with twist resulting from the increase in the cohesion of fibers as the twist is increased. The second effect is a decrease in yarn strength with twist resulting from a decrease in the effective contribution to the axial loading of the yarn due to fiber obliquity. Thus, the curve shown in Figure 7.7 may be divided into two sections (Figure 7.8): (i) a low twist region in which the effect of fiber cohesion outweighs that of obliquity, giving rise to an increase in strength, and (ii) a high twist region in which further increase in cohesion no longer produces an increase in strength because of the overwhelming effect of fiber obliquity.

The twist level used can influence a number of fabric characteristics. These include: fabric hand, and skew. High or low levels of twist may be required depending on the type of fabric produced and its desirable characteristics. Highly twisted yarns are "lively" and tend to untwist (or snarl). Consequently, fabrics made from these yarns will possess a lively handle. This effect is utilized in producing crepe yarns (TM = 5.5- 9.0), which are used to produce crepe surface cloth. When soft fabrics are desirable (e.g. knit shirts), a low level of twist is required. Low twist level is also required to minimize fabric skew. In general, the higher the level of twist in the yarn the greater the tendency for the knit fabric to skew or torque.
Yarn Diameter
The use of linear density to express the yarn fineness provides a convenient and a practical approach for characterizing this important characteristic. All machines in the fiber-to-yarn conversion system are set on the basis of the linear density of fiber strands. In certain applications, however, yarn fineness expressed in diameter or thickness provides more useful information. For example, determining the structural features of a fabric (e.g. cover factor, yarn crimp, etc.) requires a prior knowledge of yarn diameter. It is important, therefore, to measure yarn diameter or to provide an estimate of its value. In this section, we discuss methods for estimating yarn diameter.
Theoretically, equation 7.8, introduced earlier, provides a general expression of yarn radius as a function of the linear density and the volumetric density of the yarn. For direct count system (say, tex), this general relationship will be as follows:

For indirect systems (say, cotton count), the general expression of yarn diameter is as follows:

The above expressions indicate that the value of yarn diameter mainly depends on the linear density or yarn count, tex or Ne, and the volumetric density of yarn, p. As indicated earlier, volumetric density describes the degree of compactness of fibers in the yarn structure. This means that yarn twist will have a significant effect on yarn diameter.
Yarn Diameter Formula
In practice, yarn diameter is typically estimated using empirical formula. One of the most commonly used expressions for estimating yarn diameter is that developed by Peirce in 1937 (see Table 7.4). In this expression, yarn density was assumed to be 1.1 g/cm3. In a recent study, El Mogahzy et al (1993) developed empirical expressions for estimating the diameters of ring-spun, rotor-spun, and MJS airjet spun yarns. These expressions (also given in Table 7.3) were developed based on extensive microscopical testing of actual yarn thickness of the three yarn types using a wide range of yarn count, and twist levels.
The formulae shown in Table 7.3 indicate that yarns made from different spinning systems and of equal nominal count will exhibit different values of yarn diameter. This is a result of the difference in fiber arrangement and fiber compactness of different yarn types. For example, a ring-spun yarn and a rotor-spun yarn of cotton count 20's will have estimated diameters of 0.253 mm, and 0.275 mm, respectively. The higher value of rotor-spun yarn diameter indicates that it is bulkier than the ring-spun yarn.

We should point out that the formula for ring-spun yarn developed by El Mogahzy et al (1993) tend to produce a value of yarn diameter that is slightly higher than that estimated by Peirce equation. As shown in Figure 7.9, the difference between the two estimates decreases as the yarn becomes finer. The main reason for the difference was due to discrepancy in the value of yarn density, particularly in the coarse to medium range of yarn count. Using a combination of capacitive and optical measures of different yarns, we found that the density of cotton ring-spun yarns can range from 0.85 to 1.2 g/cm3 depending on the spinning system, fiber characteristics, and structural parameters (count and twist).

The Importance of Yarn Diameter
As indicated above, yarn diameter is used to estimate fabric structural parameters such as width, and cover factor. Since thousands of ends or wales are presented side-by-side in the woven or the knit fabrics, a slight change in yarn diameter can result in a substantial change in the overall cover factor of fabric. The effect of yarn diameter on the geometrical features of fabric structure can be realized through examination of the equations developed by Peirce to determine the cover factor of woven fabric (Peirce, 1937), or the equations developed by Munden (1963, 1967) to determine the tightness factor of plain weft knitted structures.
In the context of fiber-to-yarn engineering, yarn diameter is certainly a major design criterion. Factors affecting yarn diameter are essentially those that affect yarn density or fiber compactness. As we indicated earlier, fiber properties that are expected to influence fiber compactness include: fiber fineness, fiber stiffness, fiber length, and fiber crimp. In general, coarse and stiff fibers will result in bulkier or thicker yarn than fine and flexible fibers (Stout, 1958). In other words, as the fiber becomes coarser (higher denier, or millitex), yarn density becomes smaller, leading to an increase in yarn diameter, although the count of yarn remains unchanged.
Zurek (1961), one of the leading scientists in yarn structure, explains the above phenomenon on the ground that coarser or more rigid fibers have higher resistance to bending, while twisted into yarns, than finer or more flexible fibers; hence, the radius of their curvature is longer. Only movement of the fiber away from yarn axis can cause the increase of radius. On the same ground, fiber length also affects yarn density and consequently yarn diameter. For a given yarn count and at the same twist factor, the larger the fiber length, the higher the yarn density, and the smaller the yarn diameter.
In theory, fiber compactness may be characterized by two main categories of fiber arrangement in the yarn cross-section (Hearle et al, 1969): (i) the open-packed structure, and (ii) the closed-packed structure. These are illustrated in Figure 7.10. In the opened-packed structure, fibers lie in layers between successive concentric circles. The first layer is a single core fiber around which six fibers are arranged so that all are touching; the third layer has twelve fibers arranged so that the fibers first touch the circle that circumscribes the second layer; additional layers are added between the successive circumscribing circles. In the closed-packed structure, all fibers touch each others which gives rise to a hexagonal array of fibers in the yarn cross section. In practice, fiber packing may deviate largely from these idealized forms. This deviation may be attributed to a number of factors including: non-circularity of fibers, dimensional variability, the relaxation and coherence of fibers in the yarn structure, and the effect of twist. The last factor is explained on the ground that twist causes the development of tangential and radial forces, which result in fiber migration and binding of fibers together.

Yarn Strength
Yarn strength is considered as one of the main criteria characterizing yarn quality. Indeed, no other yarn characteristic has received more investigative attention than yarn strength. Most of the studies dealing with yarn strength focused on developing models characterizing yarn strength as a function of structural parameters and fiber attributes. Many of these models revealed a great deal of information about the complex nature of yarn strength. In fact, the interpretation of the strength-twist relationship discussed earlier stems from existing models describing the effect of twist on yarn strength. In recent years, interest in modeling yarn strength with respect to relevant fiber attributes has increased as a result of the revolutionary development of fiber testing and information technology, and the introduction of new spinning technologies.
Despite the numerous studies of yarn strength, no universal model exists today that can fully explore or predict the mechanical behavior of staple-fiber yarn under tensile loading, from the progressive fiber
assistance to the rupture mode. This is primarily due to the overwhelming stochastic nature of spun yarns making it very difficult to achieve a complete resolution of the different factors influencing yarn strength.
Our interest in modeling yarn strength stems from the fact that fiber-to-yarn modeling is a basic phase of fiber-to-yarn engineering. Empirical models for predicting yarn strength and other yarn characteristics can be developed within the boundaries imposed by a given textile process. These models can be verified not only through a sound database, but also on physical basis.
Practical Parameters Describing Yarn Strength
In chapter 6, we discussed the concept of load-elongation (or stress-strain) curve and the different parameters that can be derived from it. This concept basically holds for any material subject to tensile loading including textile yarns. Accordingly, the parameters associated with the curve (e.g. breaking stress, toughness, modulus, etc) can be used for characterizing yarn strength. The shape of the curve, however, varies widely depending on many factors including: yarn type (ring, rotor, or airjet), twist level, and yarn texture.
In practice, the strength of staple fiber yarn is commonly described using the following parameters:
· Skein strength
· Count-strength product (CSP)
· Single-end strength
· Strength irregularity (C.Vstrength%)
Yarn Skein Strength
The skein strength is typically measured by winding a 120-yard skein on a wrap reel. The yarn is then removed from the reel and tested in the form of several revolutions of parallel threads using a pendulum tester at a constant rate of traverse. When the specimen is subjected to tensile loading, all threads will resist the loading until a break occurs in one of the threads (the weakest point). The remaining unbroken threads will then support the skein until a second thread breaks. This process continues through a succession of thread breaks until a total failure occurs. It is believed, therefore, that the skein strength test provides a combined measure of the strength of a composite specimen of yarns and the inter-yarn friction. The parameter obtained from this test is called the skein or lea strength expressed in pounds.
The skein strength test is commonly accompanied by a yarn count test in which the same test specimen is weighted to determine the cotton count. The count-strength product known as the CSP provides a strength measure commonly known as the skein-break factor (Ne.lb). In practice, this measure is used more commonly than the absolute value of skein strength. Typical values of skein break factor for different yarns are given in Table 7.4. These values are based on yarn data corresponding to cotton U.S. crops 1990 and 1991.The wide range of CSP values is, therefore, a result of the wide range of values of fiber characteristics used in the make of the yarns.

Single-End Strength
The single-end strength represents a more fundamental parameter than the skein strength. Using modern tensile testers (e.g. Uster TensoRapid® ), strength parameters can be obtained at a constant rate of extension of 5 m/min and a gauge length of 50 cm. These parameters include: breaking load, breaking elongation, load-elongation (or stress-strain) curve, yarn tenacity, yield stress and strain, specific work of rupture, and tensile modulus. Typical values of strength parameters of different types of cotton yarns and at different values of yarn count are listed in Tables 7.5 through 7.9. These tables are modified from the Uster statistics®, 1997. Another tensile tester, also developed by Uster, is called the TensoJet®. This tester operates at a very high rate of extension (400 m/min). Using this tester, up to 30,000 tests per hour can be performed. This tester allows measuring strength variability from a large number of breaks.
Strength Irregularity (C~V~trength %)
Similar to count variability, strength irregularity is commonly defined by the coefficient of variation of yarn strength:

The importance of strength irregularity lies in the fact that during processing (warping, dyeing, weaving or knitting), the incident of breakage often occurs at the weakest points of the yarn. Knowledge of the extent of variability in yarn strength will permit estimation of the strength of the weakest points. For example, suppose that the mean strength of a ring-spun yarn of count 20's is 18 cN/tex, and the C.V% of yarn strength is 8%. From the above equation, the standard deviation of strength is 6 = 8x18/100 = 1.44 cN/tex. Typically, yarn strength as a variable follows a normal distribution. One of the basic features of the normal distribution is that the total relative frequency (or the area under the curve) between µ ± 36 is about
99.74%. As shown in Figure 7.11, it follows that this yarn will have weak points of strength values as low as 13.7 cN/tex, which is only 76% of the mean strength.



The Importance of Yarn Strength
The importance of yarn strength can be realized in all stages of processing from spinning to finished fabric manufacturing. In any spinning technique, yarn strength represents a crucial parameter, which determines the performance of spinning. For instance, an endsdown in ring spinning is often a result of the failure of the yarn to withstand a high peak of spinning tension. This failure results from a weak portion of the yarn.
The strength-twist relationship is considered to be a characteristic curve of the spinning performance that must be established to produce a high strength yarn. As indicated earlier, fiber properties such as strength, length, fineness, and friction play a vital role in determining this relationship.
During yarn preparation for weaving, the yarn is subject to continuous tension as a result of the repeated winding and unwinding necessary for weaving preparation. This tension should be within the elastic boundaries of the yarn to avoid permanent deformation. During dyeing or sizing, the yarn is subjected to chemical treatments that can alter its mechanical behavior. For example, the sizing process results in an inevitable reduction in yarn elongation and yarn flexibility. It is important, therefore, to examine the modulus and the elongation profiles of yarn during weaving preparation.
During the weaving process, thousands of yarns are simultaneously subject to continuous cyclic loading, which is a basic necessity for the interlacing actions required to make cloth. Weaving peak tension may reach levels exceeding 35% of the average breaking force of the yarn. Both tension variation, and yarn strength variation are expected. A single yarn may break when it exhibits a level of strength that is lower than the weaving tension at some points of the yarn. When a maximum tension coincides with a minimum strength point of the yarn, failure of yarn to withstand the tension will occur. This failure may result in an end breakage and a complete stop of the weaving process.
During knitting, the yarn is subject to tension, which may reach levels of more than 30% of the average breaking force of the yarn. Again, both knitting tension and yarn strength exhibit variability. Accordingly, the failure of yarn to withstand knitting tension may occur in the same fashion described for weaving. A yarn break during knitting will have an adverse effect not only on the machine efficiency but also on the fabric quality.
The stress-strain behavior of yarn is a critical factor in determining the mechanical behavior of fabric under different modes of deformation (e.g. tension, bending, and shear). In general, a strong yarn will make a strong fabric, and a stiff yarn will result in a fabric of poor comfort characteristics. An optimum combination of strength and flexibility can be achieved through many options including a proper level of twist, and a judicious choice of fiber attributes.
Yarn Evenness and Imperfections
The evenness, or regularity of a fiber strand (e.g. sliver, roving, or yarn) is a measure of the extent of uniformity in the strand thickness along its length. Imperfections represent abnormal incidents exceeding in their forms the expected variation in the thickness of a fiber strand. As shown in Figure 7.12, these include thin places, thick places, and neps.
The reference method of evenness and imperfection analysis is obviously the microscopic method. However, the large sample of yarn required to obtain reliable microscopic information makes this method time-consuming, particularly in a practical environment. Alternatively, we may take a long fiber strand, cut it into portions of equal length, and weigh each portion. The thickness variation can then be determined from the variation in the weight per unit length as shown in Figure 7.13. This method is called the "cut and weight" method and it is used as the basis for the more advanced capacitive method commonly used by most textile mills.


Methods of Evenness Testing
There are many methods that can be used for testing the evenness of a fiber strand. These include (Slater, 1986, Walker, 1950, Townsend et al, 1951):
· The capacitive method
· The optical method
· The pneumatic method
· The acoustic method, and
· The mechanical method
The capacitive method utilizes a capacitor (or an electrode). When a non-conductive material (such as a fiber strand) enters the field of the electrode, a capacitance change occurs. The variability in capacitance is used to indicate the variability in the mass of the fiber strand. The main element in the capacitive method is the detecting electrode. This consists of a pair of metal plates, acting as an air-spaced capacitor. The capacitive method is utilized in the popular Uster® evenness tester. A critical assumption underlying the use of the capacitive method is that the relationship between mass and capacitance change is linear. If the fiber/air ratio is increased beyond a certain limit, the electrode becomes overloaded and this relationship becomes non-linear. In this regard, a fiber/air ratio of 40% or less is recommended. Other limiting features of the capacitive method include the requirement of a rounded fiber strand, the necessity of keeping the strand well away from both plates or be in constant contact with one of them, and the high sensitivity of the method to relative humidity.
In the optical method, a light source is directed onto a fiber strand, and the mass per unit length of the strand is detected by either optical extinction or optical reflection. In case of the optical extinction, the shadow cast is taken to be proportional in area to the mass of the fiber strand in the test zone. In case of optical reflection, the fiber strand is directly illuminated; when a normal strand is in the test zone, no reflection is detected; abnormalities such as fluffs, loops and protruding fibers reflect light, which can be measured electrically. Optical principles are utilized in the Uster® tester, and the Zewigle EIB system. The primary limiting factor of the optical method in measuring the evenness of a fiber strand is its sensitivity to the geometrical profile of the strand. Irregular cross sections are likely to be presented to the light source in preferential direction of alignment.
In the pneumatic method, the fiber strand is passed through an orifice or a narrow tube, into which an air stream is being forced. The evenness of the fiber strand is then measured by the variation in the rate of airflow resulting from mass variation. Limiting factors of this technique include the non-linear relationship between the airflow rate and the mass of fiber strand, and the high sensitivity to atmospheric conditions (humidity and temperature). This method has been used in association with autolevelling systems (evenness control system) of fiber strands during carding.
In the acoustic method, the fiber strand moves through a sound field between a generator and a pick-up device. The time taken for sound waves to move across the gap is measured electronically. The change in this transit time is believed to correspond to the change in the cross-sectional dimensions of the fiber strand. This method has the advantage of being insensitive to moisture change. Some instrument developers have used this principle for measuring sliver uniformity during carding and drawing.
In the mechanical method, the irregularity of a fiber strand is detected using a mechanical feeler, which senses the mass variation of a fiber strand as it passes through a pair of drafting roller. It is normally utilized in conjunction with autoleveling systems.
Another important irregularity parameter is the so-called "limiting irregularity". This parameter theoretically provides an irregularity measure of a fiber strand in which fibers are arranged in a completely random fashion. In practical terms, it implies irregularity under best machine conditions. The limiting irregularity, C.V%limit , is simply defined by:

Equation 7.14 indicates that as the number of fibers per yarn, or strand, cross-section increases, the limiting irregularity decreases. This may be explained on the ground that the increase in the number of fibers creates a compensating or a doubling effect that reduces the irregularity in yarn cross-section.
In practice, the concept of limiting irregularity can be used to estimate the partial effect of process-added variability on the overall irregularity. In this regard, the Uster evenness tester can provide the so-called "irregularity index" defined by the following equation:
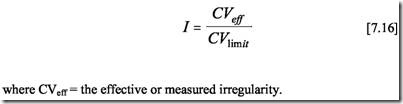
The concept underlying the utilization of an irregularity index is that every process will inevitably add variability to the fiber strand. This added variability is a result of the limited capability of existing processes to maintain a perfectly random distribution of fibers in the strand cross-section, and along the strand length. In addition, mechanical defects such as improper fiber control and draft roller eccentricity adds a periodic component to the variability in the fiber strand. The irregularity index, I, compares the limiting variability to the total measured variability of the fiber strand. In the ideal situation where no process-added irregularity exists, both the measured and the limiting irregularities will be theoretically equal; in this case, the irregularity index will be equivalent to unity. In actual processing, however, the measured irregularity will exceed the limiting irregularity, and a value of I greater than one will be expected.
Yarn Imperfections
Staple-fiber yarns usually exhibit 3 main types of imperfections: thin places, thick places, and neps. In the USTEO evenness tester, thin and thick places refer to imperfections that are within the measuring sensitivity range (f 100% with respect to the mean value of yarn cross-sectional size). Figure 7.15 shows a relative frequency diagram showing the yarn sensitivity range. Typically, thin and thick places can be of up to one-inch length. Neps are classified as the yarn imperfections, which may exceed the f 100% limit. They are typically of 3 to 10 mm length. Typical values of Uster imperfections are listed in Tables 7.12 through 7.14. Thick places exceeding the 100% limit are determined using the so-called Classimat method.



Yarn Surface Integrity
The critical importance of yarn surface integrity stems from the fact that despite the advanced spinning technology that we witness today, the yarn as spun can not be woven or knitted without some form of treatment to enhance its surface integrity. Millions of dollars are spent every day to apply chemicals to the yarn surface so that it can flow smoothly through the weaving process. These chemicals provide a temporary function and are later disposed or partially recycled. The cost of these chemicals and their byproduct environmental effects clearly justify extensive research in the area of yarn surface to seek ways to improve the inherent surface structure of spun yarns.
In practice, yarn surface integrity is typically characterized by two main parameters: abrasion resistance and hairiness. Abrasion is generally defined as the wearing away of any part of the material by rubbing against another surface. Accordingly, the measuring principle of abrasion resistance is normally based on placing a number of parallel threads under a predetermined initial tension, and subjecting these threads to an abrasive solid surface moving (or rotating) at a constant speed. This will exert a constant abrasive force, which continues to act on the yarn surface until the yarn is finally worn out. The abrasion resistance is commonly expressed by the number of abrasive cycles required to break the yarn.
Testing of abrasion resistance of staple fiber yarns is often associated with a lack of repeatability of test results. This is largely attributed to the complex variable nature of yarn surface and to the presence of fiber loops and hairs protruding from the surface.
Yarn hairiness may generally be defined as the extent of hairs protruding from the yarn body. Two methods are currently used for measuring yarn hairiness: (i) the hair count method, and (ii) the hair length method. In the first method, fibers protruding from the yarn surface are counted by projecting the fiber shadow onto phototransistors. This method is utilized in the Zweigle® hairiness measuring device, which provides values of the number of hairs per meter; hairs extending over lengths from 1 mm to 25 mm can be counted. Obviously, the maximum number of hairs will be detected at the closest distance to the yarn body (1 mm).
In the second method, the measuring field is formed by homogenous rays of parallel light; if a yarn lies in this field, only those rays of light scattered by the fibers protruding from the yarn body are detected. This method is utilized in the USTE~® evenness tester. Hairiness in this case is defined by the index H, which is defined as the total length (in cm) of all protruding hairs with reference to a sensoring length of 1 cm. For example, a hairiness value H = 5 will correspond to a total protruding fiber length of 5 cm per 1 cm sensing length. Typical values of Uster hairiness are shown in Tables 7.15 and 7.16.
