At present the significant direction of researches on the processes and systems of spinning technology is to find out a scientific method of dynamic simulation. This can be done by the application of the theory of random function. The principle lies on the conception of technological system as a stationary, linear dynamic system. The aim is to determine the modulus of the relative transfer function and subsequent technological analysis. The acts can be stated as to determine the influence of technological factors on the equalization effectiveness to a given spinning system and also to determine the conditions for improving the quality of the resulting linear fiber products (for example, sliver, roving, and yarn) from the point of view of mass irregularity.
It is necessary to point out that a procedure of dynamic simulation can solve the question of transformation through the spinning systems. The problem of transformation can also be solved with the help of determination of quadratic mass irregularity of short sections of the linear fiber products on the basis of autocorrelation function of mass irregularity.
Choice of the technological application of dynamic simulation
As an example of the method of dynamic simulation as well as process of determination of quadratic mass irregularity of short section of linear fiber product, we can discuss about the following technological applications.
- flat card with a drafting mechanism [ 1 ] – evaluation of the leveling effectiveness - a system of successive and combined doubling[2]- levelling effectiveness,
- the resulting quadratic unevenness, the technological causes of an increased levelling
effectiveness, the effect of replacement of spinning rotors with the increasing
frequency of revolution
- system of cyclic doubling [3] – the resulting quadratic unevenness, the influence of the collecting surface diameter of the rotor, requirements of the fiber flow taken over by the collecting surface
- the separating device of the OE- spinning system [4] – influence of the structure of the mass irregularity in the fiber flow
The main problem in the application of method of dynamic simulation is the process of determination of transfer function, subsequently modulus of relative transfer function. Relevant determination is possible with theoretical or experimental process. Dynamic model detailing transformation of mass irregularity comes from two basis aspects of a given technological problem. With these two basis aspects of the specified research problems, the following possibilities can be stated:
a) the quantities expressing the character of the characteristic property of a fiber product: these can be
- deterministic or
- random
b) the mechanical and physical action defining the character of the technological environment can be characterized as
- deterministic or
- random
In the dynamic model, the character of fiber product from point of view of mass of short sections of linear fiber product is random. The character of technological environment i.e., system of transformation is determined by the simplifying condition of processes.
Modulus of relative transfer function for OE – rotor spinning system
The concept of OE – rotor-spinning system as a dynamic system including the division into partial dynamic system is stated in [5]. An equation expressing the modulus of relative transfer function for system of cyclic doubling in spinning rotor process can be found in [5]. The main content of the present work is the presentation of results of experimental procedure for determination of the modulus of relative transfer function for OE – rotor-spinning system and the possibility of use of the apparatus Uster Tester 4 – SX is also explored. The spectrograms obtained from the above-mentioned apparatus may be directly used for determination of the modulus of relative transfer function for the given system. In principle, this acts about record harmonic components of mass irregularity depends on the wavelength. Instead of amplitude of the harmonic components, the quadratic mass irregularity (CV) of the harmonics components is plotted along the y-axis. This is directly proportion to the relative amplitude, so the modulus of the relative transfer function can be directly expressed as a ratio between the CV values of the corresponding harmonic components. It is important to obtain the CV values correctly in order to obtain correct values of the modulus, hence efficient application.
Results of experimental determination of the modulus of relative transfer function for the OE–rotor-spinning system are given below.
Interpretation of results and conclusion
The method of determination of the modulus of the relative transfer function for a given spinning system with the use of spectrograms obtained from the apparatus Uster Tester 4-SX makes it possible to obtain a function that characterizes transformation of the structural mass irregularity of the input fiber product (sliver) on the structural mass irregularity of the resulting or output fiber product (yarn). It offers a picture on the components of the OE-spinning system, which is important for preparation of sliver with optimal structural mass irregularity.
In the given example of OE-rotor-spinning system, it is known that there exits two very contradictory effects from point of view of mass irregularity, that is, the opening system highly increases the mass irregularity and the cyclic doubling reduces it. Globally,
the OE-rotor-spinning system is known as a system of very high draft (in the given example draft is P = 236) and that matches with the resulting modulus of the relative transfer function. It is evident, that with reference to the very high draft, given to an OE-rotor spinning system, thanks to the system of cyclic doubling, that decreases the mass irregularity!
A new philosophy, which uses the principle of dynamic simulation opens new directions of research for analysis of structural mass irregularity with a view to different consequential optimization of different spinning systems from the point of view of mass irregularity of the resulting fiber product.
P.Ursíny,R.K.Nag
Department of Textile Mechanical Technologies, Faculty of Textiles,
Technical University of Liberec, Hálkova 6, Liberec 1, 461 17, Czech Republic


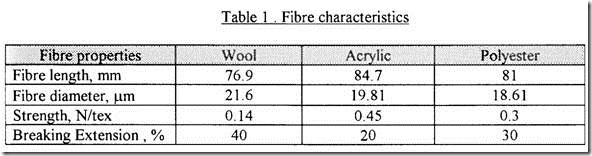





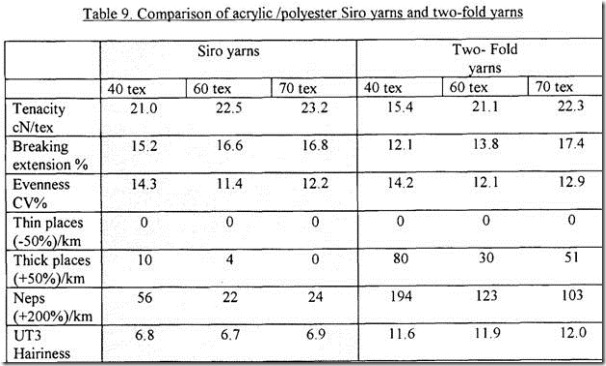










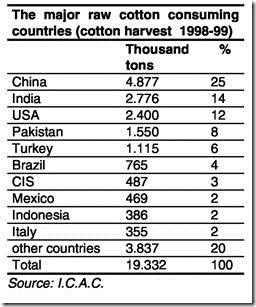






![clip_image020[3] clip_image020[3]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEiOmkzWbN2_ZkoylEt_dEHEU-N8nVO443oLzkTpfmG7E13_de03mFmmSiToxKjFT1lgD3mki7LxW8e1ZiS31pAJ5A0VBosjjumpCC6FNXGaliguURWuOHHy0HqlOsyL8rOb3o0eW3i6ZUb6/?imgmax=800)
![clip_image022[3] clip_image022[3]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEjY4pME6eSvtDQIVYhcQV5zDqMCePnGwxb65eB_saR5UA44y8M7DGq8l6SSF1dJhN1xJE6rkKuGX_7sm8K-BrBTRHG9NhWL56j9i57Rg1iJl4P8ZklCvDGgaTxjp7VM36J4A-UY05N6UQ1a/?imgmax=800)


![clip_image024[3] clip_image024[3]](https://blogger.googleusercontent.com/img/b/R29vZ2xl/AVvXsEgF03hHzowmoNGMXZnbro3IoDNHT-yyiggDvRxXlmyEiD8tNABdTak8E4rQUD6CHZVuS6LlmPnvQUUGhunmUlo9XDbChhP30X9dvSTOmA3tHa-uz_P9-jws1uM12hPcGUcpV_cpzgZEzEkm/?imgmax=800)